Basics & Formulas Ch-3 Class11 | Trigonometry

TRIGONOMETRY : CLASS 11 : CHAPTER 3

Different systems of angles, radian measure, degree, AB and CD formulas,  transformations of angles in different quadrants and solution of trigonometric equations

Trigonometry:

It is the branch of mathematics in which we deal with the relation between the angle and sides of the right triangle.

Table 1

	0o	30o	45o	60o	90o	180o	270o	360o
Sin	0	1/2	\[1/\sqrt{2}\]	\[\sqrt{3}/2\]	1	0	-1	0
Cos	1	\[\sqrt{3}/2\]	\[1/\sqrt{2}\]	1/2	0	-1	0	1
Tan	0	\[1/\sqrt{3}\]	1	\[\sqrt{3}\]	\[\infty\]	0	\[\infty\]	0
Cot	\[\infty\]	\[\sqrt{3}\]	1	\[1/\sqrt{3}\]	0	\[\infty\]	0	\[\infty\]
Sec	1	\[2/\sqrt{3}\]	\[\sqrt{2}\]	2	\[\infty\]	-1	\[\infty\]	1
cosec	\[\infty\]	2	\[\sqrt{2}\]	\[2/\sqrt{3}\]	1	\[\infty\]	-1	\[\infty\]

DIFFERENT SYSTEMS OF ANGLES

There are mainly three systems of measuring angles:

1) Centesimal System,  2) Sexagesimal System,  3) Circular System.

CENTESIMAL SYSTEM

 

SEXAGESIMAL SYSTEM

 

CIRCULAR SYSTEM

Radian Measure:- Angle made by an arc of unit length in a circle of unit radius is called one radian

 

 

RELATION BETWEEN DEGREE AND RADIAN

Note : In order to convert degree measure into radian measure, we should multiply the angle in the degree measure by 

In order to convert a radian measure into a degree measure we should multiply the angle in the radian measure by  

MOVEMENT OF ANGLE IN DIFFERENT  QUADRANT

SIGN OF TRIGONOMETRIC RATIOS IN DIFFERENT QUADRANT

TRIGONOMETRY-CBSE Mathematics

COMPLETE  TRANSFORMATION OF ANGLES

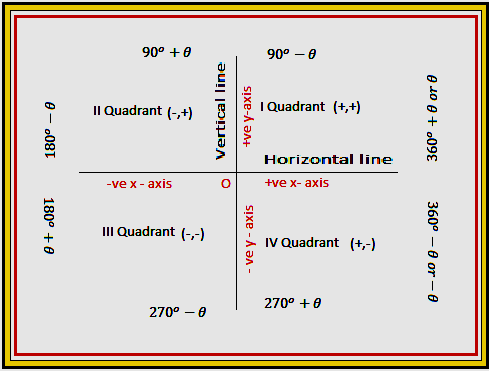
Trigonometric Transformations at 90o (I and II Quadrant)
sin(90 - θ)	+cosθ		sin(90 + θ)	+cosθ
cos(90 – θ)	+Sinθ		cos(90 + θ)	-Sinθ
tan(90 - θ)	+cotθ		tan(90 + θ)	-cotθ
cot(90 - θ)	+tanθ		cot(90 + θ)	-tanθ
sec(90 - θ)	+cosecθ		sec(90 + θ)	-cosecθ
cosec(90 - θ)	+secθ		cosec(90 + θ)	+secθ

Trigonometric Transformations at 180o  (II and III Quadrant)
sin(180 - θ)	Sin θ		sin(180 + θ)	-Sin θ
cos(180 – θ)	-Cos θ		cos(180 + θ)	-Cos θ
tan(180 - θ)	-tanθ		tan(180 + θ)	+tanθ
cot(180 - θ)	-cotθ		cot(180 + θ)	+cotθ
sec(180 - θ)	-secθ		sec(180 + θ)	-secθ
cosec(180 - θ)	cosecθ		cosec(180 + θ)	-cosecθ

Trigonometric Transformations at 270o  (III and IV Quadrant)
sin(270 - θ)	-cosθ		sin(270 + θ)	-cosθ
cos(270 – θ)	-Sinθ		cos(270 + θ)	+Sinθ
tan(270 - θ)	+cotθ		tan(270 + θ)	-cotθ
cot(270 - θ)	+tanθ		cot(270 + θ)	-tanθ
sec(270 - θ)	-cosecθ		sec(270 + θ)	+cosecθ
cosec(270 - θ)	-secθ		cosec(270 + θ)	-secθ

Trigonometric Transformations at 360o  (IV and I Quadrant)
sin(360 - θ)	-Sin θ		sin(360 + θ)	+Sin θ
cos(360 – θ)	+Cos θ		cos(360 + θ)	+Cos θ
tan(360 - θ)	-tanθ		tan(360 + θ)	+tanθ
cot(360 - θ)	-cotθ		cot(360 + θ)	+cotθ
sec(360 - θ)	+secθ		sec(360 + θ)	+secθ
cosec(360 - θ)	-cosecθ		cosec(360 + θ)	+cosecθ

Trigonometric Transformations at 0o   (IV and I Quadrant)
sin(0 - θ)	-Sin θ		sin(0+θ)	+ Sin θ
cos(0 - θ)	+Cos θ		cos(0+θ)	+ Cos θ
tan(0 - θ)	-tanθ		tan(0+θ)	+ tanθ
cot( 0-θ)	-cotθ		cot(0+θ)	+ cotθ
sec( 0-θ)	+secθ		sec(0+θ)	+ secθ
cosec( 0-θ)	-cosecθ		cosec(0+θ)	+ cosecθ

STANDARD ANGLES IN DEGREE MEASURE AND RADIAN MEASURE

Table 2

Degree Measure	30o	45o	60o	90o	180o	270o	360o
Radian Measure	\[\frac{\pi }{6}\]	\[\frac{\pi }{4}\]	\[\frac{\pi }{3}\]	\[\frac{\pi }{2}\]	\[\pi\]	\[\frac{3\pi }{2}\]	\[2\pi\]

TRIGONOMETRIC FORMULAS WITH COMPOUND ANGLE

Sin(A + B) = SinACosB + CosASinB

Sin(A - B) = SinACosB - CosASinB

Cos(A + B) = CosACosB - SinA SinB

Cos(A - B) = CosACosB + SinA SinB

TRIGONOMETRIC FORMULAS WITH MULTIPLE ANGLE

\[Sin2\theta =2Sin\theta\; Cos\theta \; \; \; OR\; \; \frac{2tan\theta }{1+tan^{2}\theta }\]\[Cos2\theta =Cos^{2}\theta -Sin^{2}\theta,\; \; or\: \: 2Cos^{2}\theta -1,\; \; or\; \; 1-2Sin^{2}\theta \; \; or\; \; \frac{1-tan^{2}\theta }{1+tan^{2}\theta }\]\[tan2\theta =\frac{2tan\theta }{1-tan^{2}\theta }\]\[Sin3\theta =3Sin\theta -4Sin^{3}\theta\]\[Cos3\theta =-\left ( 3Cos\theta -4Cos^{3}\theta \right )\: \: or\: \: \left ( 4Cos^{3}\theta -3Cos\theta \right )\]\[Tan3\theta =\frac{3tan\theta -tan^{3}\theta }{1-3tan^{2}\theta }\]\[Sin^{2}\theta =\frac{1-cos2\theta }{2},\: \: \: Sin^{2}4\theta =\frac{1-cos8\theta }{2}\]\[Cos^{2}\theta =\frac{1+cos\: 2\theta }{2},\: \: \: Cos^{2}\: 4\theta =\frac{1+cos\: 8\theta }{2}\]\[tan^{2}\; \theta =\frac{1-cos2\theta }{1+cos2\theta },\:\: \: \: \: tan^{2}\: 4\theta =\frac{1-cos\: 8\theta }{1+cos\: 8\theta }\]

TRIGONOMETRIC FORMULAS WITH SUB-MULTIPLE ANGLE

\[Sin\theta =2\: sin\: \frac{\theta }{2}\: cos\: \frac{\theta }{2},\:\: or\: \:\; \frac{2tan\frac{\theta }{2}}{1+tan^{2}\frac{\theta }{2}}\]\[Cos\theta =Cos^{2}\; \frac{\theta }{2} -Sin^{2}\;\frac{\theta }{2},\; \; or\; \; 2Cos^{2}\; \frac{\theta }{2} -1,\; \; or\; \; 1-2Sin^{2}\; \frac{\theta}{2} \; \; or\; \; \frac{1-tan^{2}\; \frac{\theta}{2} }{1+tan^{2}\; \frac{\theta}{2} }\]\[Tan\theta =\frac{2tan\frac{\theta }{2}}{1-tan^{2}\frac{\theta }{2}}\]

\[Sin^{2}\; \frac{\theta}{2} =\frac{1-cos\; \theta }{2},\: \:\; \; \: Cos^{2}\; \frac{\theta}{2} =\frac{1+cos\: \theta }{2}\]\[tan^{2}\; \frac{\theta}{2} =\frac{1-cos\theta }{1+cos\theta },\]

A, B FORMULAS:-

\[2SinA\: CosB=Sin(A+B)+Sin(A-B)\]\[2CosA\: SinB=Sin(A+B)-Sin(A-B)\]\[2CosA\: CosB=Cos(A+B)+Cos(A-B)\]\[2SinA\: SinB=-Cos(A+B)+Cos(A-B)\]

C,D FORMULAS:- 

\[SinC+SinD=2Sin\left (\frac{C+D}{2} \right )Cos\left ( \frac{C-D}{2} \right )\]\[SinC-SinD=2Cos\left (\frac{C+D}{2} \right )Sin\left ( \frac{C-D}{2} \right )\]\[CosC+CosD =2Cos\left (\frac{C+D}{2} \right )Cos\left ( \frac{C-D}{2} \right )\]\[CosC-CosD=-2Sin\left (\frac{C+D}{2} \right )Sin\left ( \frac{C-D}{2} \right )\]

SOME SPECIAL FORMULAS

\[Tan\left ( 45+\theta \right )=\frac{1+tan\theta }{1-tan\theta }\]\[Tan\left ( 45-\theta\right )=\frac{1-tan\theta }{1+tan\theta }\]\[Sin^{2}A-Sin^{2}B=Sin(A+B)Sin(A-B)\]\[Cos^{2}A-Cos^{2}B=Sin(A+B)Sin(A-B)\]

PERIODIC FUNCTIONS

All trigonometric ratios are periodic functions

\[Sin(x+2\pi )=Sin(x+4\pi )=Sin(x+6\pi )=Sin(x+8\pi )=..........\]\[Cos(x+2\pi )=Cos(x+4\pi )=Cos(x+6\pi )=Cos(x+8\pi )=..........\]\[Sec(x+2\pi )=Sec(x+4\pi )=Sec(x+6\pi )=Sec(x+8\pi )=..........\]\[Cosec(x+2\pi )=Cosec(x+4\pi )=Cosec(x+6\pi )=Cosec(x+8\pi )=..........\]\[On\; changing\; x\; to\; 2n\pi,\; sinx,\; cosx,\; secx,\; cosecx\; all\; remain\; unchanged.\]\[sinx,\; cosx,\; secx,\; cosecx\; all\; are\; periodic\; functions\; and\; their\; period\; is\; 2\pi\]\[tanx=tan(x\pm \pi )=tan(x\pm 2\pi )=tan(x\pm 3\pi )=........\]\[cotx=cot(x\pm \pi )=cot(x\pm 2\pi )=cot(x\pm 3\pi )=........\]\[tanx\; and\; cotx\; are\; periodic\; and\; their\; period\; is\; \pi\]

PRINCIPAL SOLUTION OF TRIGONOMETRIC FUNCTIONS

\[Solutions\; of\; trigonometric\; equations\; for\; which\;\; 0\leq x\leq 2\pi \; \\ are\; called\; principal\; solutions\]

PERIODIC VALUES:-

It is the least value which when added to the given function so that the value of that function remain unchanged.

TRIGONOMETRIC EQUATIONS:-

Equations involving trigonometric functions of a variable are called trigonometric equations.

GENERAL SOLUTIONS OF TRIGONOMETRIC EQUATIONS:-

\[If\; sinx=0,\; \; then\; x=n\pi\]\[If\; cosx=0,\; \; then\; x=(2n+1)\frac{\pi }{2}\]\[If\; tanx=0,\; \; then\; x= n\pi\]\[If\; sinx=siny\; \; then\; x=n\pi +(-1)^{n}y\]\[If\; cosx=cosy,\; \; then\; x=2n\pi \pm y\]\[If\; tanx=tany,\; \; then\; x=n\pi +y\]

Miscellaneous Exercise Chapter 3 Class 11

Q 8) If tanx = -4/3, and x lie in the II quadrant then find the value of sinx/2, cosx/2, tanx/2
Solution: Since angle lie in the II quadrant. Therefore 

  \[\frac{\pi }{2}<x<\pi \Rightarrow \frac{\pi }{4}<\frac{x}{2}<\frac{\pi }{2}\]⇒ Angle  x/2 lie in the first quadrant and in first quadrant sinx/2,  cosx/2 and tanx/2 all are positive  \[tanx=\frac{-4}{3}=\frac{P}{B}\]\[\Rightarrow P= 4k,\: and\: B= -3k\]\[In\: \: \Delta OAB,\; OA=\sqrt{(4k)^{2}+(3k)^{2}}=5k\]\[Now\: \: cosx =\frac{B}{H}=\frac{-3k}{5k}=\frac{-3}{5}\]\[sin^{2}\; \frac{x}{2}=\frac{1-cosx}{2}=\frac{1-(\frac{-3}{5})}{2}\]\[sin^{2}\; \frac{x}{2}=\frac{1+\frac{3}{5}}{2}=\frac{8}{2\times 5}=\frac{4}{5}\]\[sin\; \frac{x}{2}=\sqrt{\frac{4}{5}}=\frac{2}{\sqrt{5}}\]\[cos^{2}\: \frac{x}{2}=\frac{1+cosx}{2}=\frac{1-\frac{3}{5}}{2}\]\[cos^{2}\: \frac{x}{2}=\frac{2}{2\times 5}=\frac{1}{5}\]\[cos\: \frac{x}{2}=\sqrt{\frac{1}{5}}=\frac{1}{\sqrt{5}}\]\[tan\frac{x}{2}=\frac{sin\frac{x}{2}}{cos\frac{x}{2}}=\frac{2/\sqrt{5}}{1/\sqrt{5}}=2\]

Worksheet(1) for the students   (Pair the following)
Which do not have any pair write answer for them also
1) \[tan2x\]	a) \[sinx\]
2) \[\frac{tan2x}{1+tan^{2}x}\]	b) \[\frac{2tan\frac{x}{2}}{1-tan^{2}\frac{x}{2}}\]
3) \[2cos^{2}\frac{x}{2}-1\]	c) \[\frac{2tan\frac{x}{2}}{1+tan^{2}\frac{x}{2}}\]
4) \[sinx\]	d) \[\frac{2tanx}{1-tan^{2}x}\]
5) \[\sqrt{1-cos^{2}x}\]	e) \[|sinx|\]
6) \[\frac{1-tan\frac{x}{2}}{1+tan\frac{x}{2}}\]	f)\[sin2x\]
	g)\[sinx\]
7) \[sec^{2}x-1\]	h)\[cosx\]
8) \[\frac{1-tan^{2}\frac{x}{2}}{1+tan^{2}\frac{x}{2}}\]	i) \[2sin\frac{x}{2}cos\frac{x}{2}\]
	j) \[tan\left ( \frac{\pi }{4}-\frac{x}{2} \right )\]
9) \[cosAcosB+sinAsinB\]	k) \[cos(B-A)\]
10) \[tan\left (\frac{\pi }{4}+x \right )\]	l)\[cos(A+B)\]
	m) \[\frac{1+tanx}{1-tanx}\]

Answer Key : (1, d), (2, f), (3, n), (4, c and i), (5, e), (6, j), (7, tan²x), (8, h), (9, k), (10, m)

Worksheet(2) for the students   Pair the following
1) \[\sqrt{1-cosx}\]	a\[\frac{tanA-tanB}{1-tanAtanB}\]
2) \[\sqrt{1+cosx}\]	b \[4cos^{3}x-3cosx\]
3) \[\sqrt{1-sin2x}\]	c \[\frac{3tan-tan^{3}x}{1-3tan^{2}x}\]
4) \[\sqrt{1+sin2x}\]	d \[sinAcosB-cosAsinB\]
5) \[sin3x\]	e \[3sinx-4sin^{3}x\]
6) \[cos3x\]	f \[|cosx-sinx|\]
7) \[tan3x\]	g \[\left |\sqrt{2}sin\frac{x}{2} \right |\]
8) \[sin(A-B)\]	h \[|cosx+sinx|\]
9) \[tan(A-B)\]	i \[\left |\sqrt{2}cos^{2}\frac{x}{2} \right |\]

Answer Key: (1, g), (2, i), (3, f), (4, h), 5, e), (6, b), (7, c), (8, d), (9, a)

THANKS FOR YOUR VISIT

PLEASE COMMENT BELOW